Quantum Wave in a Box |
| この情報はストアのものより古い可能性がございます。 | ||||
| 価格 | 無料 | ダウンロード |
||
|---|---|---|---|---|
| ジャンル | 教育 | |||
サイズ | 11.5MB | |||
| 開発者 | Michel Ramillon | |||
| 順位 |
| |||
| リリース日 | 2017-01-01 11:55:45 | 評価 | 評価が取得できませんでした。 | |
| 互換性 | iOS 11.0以降が必要です。 iPhone、iPad および iPod touch 対応。 | |||
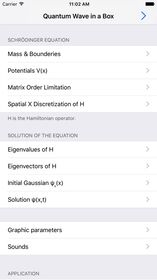
Schrödinger equation solver 1D. User defined potential V(x). Diagonalization of hamiltonian matrix. Animation showing evolution in time of a gaussian wave-packet.
In Quantum Mechanics the one-dimensional Schrödinger equation is a fundamental academic though exciting subject of study for both students and teachers of Physics. A solution of this differential equation represents the motion of a non-relativistic particle in a potential energy field V(x). But very few solutions can be derived with a paper and pencil.
Have you ever dreamed of an App which would solve this equation (numerically) for each input of V(x) ?
Give you readily energy levels and wave-functions and let you see as an animation how evolves in time a gaussian wave-packet in this particular interaction field ?
Quantum Wave in a Box does it ! For a large range of values of the quantum system parameters.
Actually the originally continuous x-spatial differential problem is discretized over a finite interval (the Box) while time remains a continuous variable. The time-independent Schrödinger equation H ψ(x) = E ψ(x), represented by a set of linear equations, is solved by using quick diagonalization routines. The solution ψ(x,t) of the time-dependent Schrödinger equation is then computed as ψ(x,t) = exp(-iHt) ψ₀(x) where ψ₀(x) is a gaussian wave-packet at initial time t = 0.
You enter V(x) as RPN expression, set values of parameters and will get a solution in many cases within seconds !
- Atomic units used throughout (mass of electron = 1)
- Quantum system defined by mass, interval [a, b] representing the Box and (real) potential energy V(x).
- Spatially continuous problem discretized over [a, b] and time-independent Schrödinger equation represented by a system of N+1 linear equations using a 3, 5 or 7 point stencil; N being the number of x-steps. Maximum value of N depends on device’s RAM: up to 4000 when computing eigenvalues and eigenvectors, up to 8000 when computing eigenvalues only.
- Diagonalization of hamiltonian matrix H gives eigenvalues and eigenfunctions. When computing eigenvalues only, lowest energy levels of bound states (if any) with up to 10-digit precision.
- Listing of energy levels and visualisation of eigenwave-functions.
- Animation shows gaussian wave-packet ψ(x,t) evolving with real-time evaluation of average velocity, kinetic energy and total energy.
- Toggle between clockwise and counter-clockwise evolution of ψ(x,t).
- Watch Real ψ, Imag ψ or probability density |ψ|².
- Change initial gaussian parameters of the wave-packet (position, group velocity, standard deviation), enter any time value, then tap refresh button to observe changes in curves without new diagonalization. This is particularly useful to get a (usually more precise) solution for any time value t when animation is slower in cases of N being large.
- Watch both solution ψ(x,t) and free wave-packet curves evolve together in time and separate when entering non-zero potential energy region.
- Zoom in and out any part of the curves and watch how ψ(x,t) evolve locally.
更新履歴
Update for iOS 16.
In Quantum Mechanics the one-dimensional Schrödinger equation is a fundamental academic though exciting subject of study for both students and teachers of Physics. A solution of this differential equation represents the motion of a non-relativistic particle in a potential energy field V(x). But very few solutions can be derived with a paper and pencil.
Have you ever dreamed of an App which would solve this equation (numerically) for each input of V(x) ?
Give you readily energy levels and wave-functions and let you see as an animation how evolves in time a gaussian wave-packet in this particular interaction field ?
Quantum Wave in a Box does it ! For a large range of values of the quantum system parameters.
Actually the originally continuous x-spatial differential problem is discretized over a finite interval (the Box) while time remains a continuous variable. The time-independent Schrödinger equation H ψ(x) = E ψ(x), represented by a set of linear equations, is solved by using quick diagonalization routines. The solution ψ(x,t) of the time-dependent Schrödinger equation is then computed as ψ(x,t) = exp(-iHt) ψ₀(x) where ψ₀(x) is a gaussian wave-packet at initial time t = 0.
You enter V(x) as RPN expression, set values of parameters and will get a solution in many cases within seconds !
- Atomic units used throughout (mass of electron = 1)
- Quantum system defined by mass, interval [a, b] representing the Box and (real) potential energy V(x).
- Spatially continuous problem discretized over [a, b] and time-independent Schrödinger equation represented by a system of N+1 linear equations using a 3, 5 or 7 point stencil; N being the number of x-steps. Maximum value of N depends on device’s RAM: up to 4000 when computing eigenvalues and eigenvectors, up to 8000 when computing eigenvalues only.
- Diagonalization of hamiltonian matrix H gives eigenvalues and eigenfunctions. When computing eigenvalues only, lowest energy levels of bound states (if any) with up to 10-digit precision.
- Listing of energy levels and visualisation of eigenwave-functions.
- Animation shows gaussian wave-packet ψ(x,t) evolving with real-time evaluation of average velocity, kinetic energy and total energy.
- Toggle between clockwise and counter-clockwise evolution of ψ(x,t).
- Watch Real ψ, Imag ψ or probability density |ψ|².
- Change initial gaussian parameters of the wave-packet (position, group velocity, standard deviation), enter any time value, then tap refresh button to observe changes in curves without new diagonalization. This is particularly useful to get a (usually more precise) solution for any time value t when animation is slower in cases of N being large.
- Watch both solution ψ(x,t) and free wave-packet curves evolve together in time and separate when entering non-zero potential energy region.
- Zoom in and out any part of the curves and watch how ψ(x,t) evolve locally.
更新履歴
Update for iOS 16.
ブログパーツ第二弾を公開しました!ホームページでアプリの順位・価格・周辺ランキングをご紹介頂けます。
ブログパーツ第2弾!
アプリの周辺ランキングを表示するブログパーツです。価格・順位共に自動で最新情報に更新されるのでアプリの状態チェックにも最適です。
ランキング圏外の場合でも周辺ランキングの代わりに説明文を表示にするので安心です。
サンプルが気に入りましたら、下に表示されたHTMLタグをそのままページに貼り付けることでご利用頂けます。ただし、一般公開されているページでご使用頂かないと表示されませんのでご注意ください。
幅200px版
幅320px版
Now Loading...

「iPhone & iPad アプリランキング」は、最新かつ詳細なアプリ情報をご紹介しているサイトです。
お探しのアプリに出会えるように様々な切り口でページをご用意しております。
「メニュー」よりぜひアプリ探しにお役立て下さい。
Presents by $$308413110 スマホからのアクセスにはQRコードをご活用ください。 →
Now loading...